When using hydraulics or hydronics in mechatronics projects the designer must know how the devices work and this implies to know the fundamentals concepts and laws of the mechanics of liquids. In the next lines we will give to the reader some formulas, tables, applications and basic concepts involving hydrotronics (*).
Pressure
a) Pressure is the amount of force for unit of area..
Formula
P = F/S
Where:
P is the pressure
F is the force
S is the area where the force is applied
b) Pressure of a column of liquid.
Formula
P = ρ x g x h
Where:
P is the pressure
ρ is the density of the liquid
g is the acceleration of gravity (9.8 m/s2)
h is the height of the column
c) Pressure in determinated depth of water:
Formula
P = h x D
Where:
P is the pressure
h is the depth of the considered point
D is the density of the water (or other liquid)
Table
Density of Water in Different Temperatures
| T (°C) | D | (g/mL) |
| 0 | 0.999 | 87 |
| 1 | 0.999 | 93 |
| 2 | 0.999 | 97 |
| 3 | 0.999 | 99 |
| 4 | 1.000 | 00 |
| 5 | 0.999 | 99 |
| 6 | 0.999 | 97 |
| 7 | 0.999 | 93 |
| 8 | 0.999 | 88 |
| 9 | 0.999 | 81 |
| 10 | 0,999 | 73 |
| 11 | 0.999 | 63 |
| 12 | 0.999 | 52 |
| 13 | 0.999 | 40 |
| 14 | 0.009 | 27 |
| 15 | 0.999 | 13 |
| 16 | 0.998 | 97 |
| 17 | 0.998 | 80 |
| 18 | 0.998 | 62 |
| 19 | 0.998 | 43 |
| 20 | 0.998 | 23 |
| 21 | 0.997 | 02 |
| 22 | 0.997 | 80 |
| 23 | 0.997 | 57 |
| 24 | 0.997 | 32 |
| 25 | 0.997 | 07 |
| 26 | 0.996 | 81 |
| 27 | 0.996 | 52 |
| 28 | 0.996 | 22 |
| 29 | 0.995 | 92 |
| 30 | 0.995 | 61 |
Table
Density of Some Liquids
(at 20o C)
| Liquid | Density (g/cm3) |
| Acetic acid | 1.049 |
| Acetone | 0.791 |
| Aniline | 1.02 |
| Benzene | 0.879 |
| Benzine | 0.68 - 0.72 |
| Chloroform | 1.489 |
| Crude Oil | 0.76 - 0.86 |
| Ethyl Alcohol | 0.79 |
| Formic acid | 1.22 |
| Glycerine | 1.26 |
| Heavy Water | 1.108 6 |
| Heptane | 0.684 |
| Hexane | 0.660 |
| Machine oil | 0.8 - 0.9 |
| Mercury | 13.55 |
| Methyl Alcohol | 0.792 |
| Milk (average) | 1.03 |
| Nitric acid | 1.51 |
| Nitroglycerine | 1.6 |
| Sea water | 1.01 - 1.03 |
| Water | 0.998 23 |
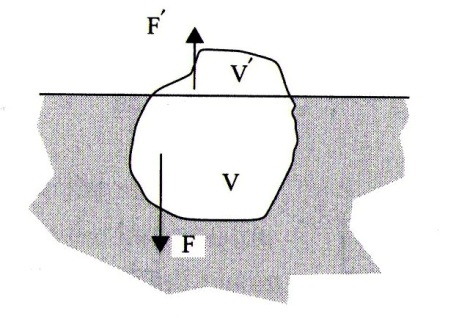
Archimedes' Principle
A body, immersed in a liquid is buoyed up by a force equal to the weight of the liquid displaced.
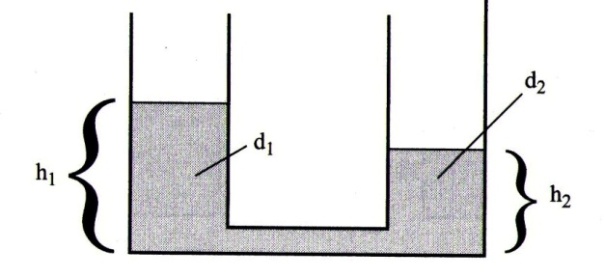
Communicating vessels
The heigths of columns in communicating vessels are inversely proportional to their densities.
Formula
h1/h2 = d2/d1
Where:
h1 and h2 are the heigths of columns
d1, d2 are the denseities of the liquids
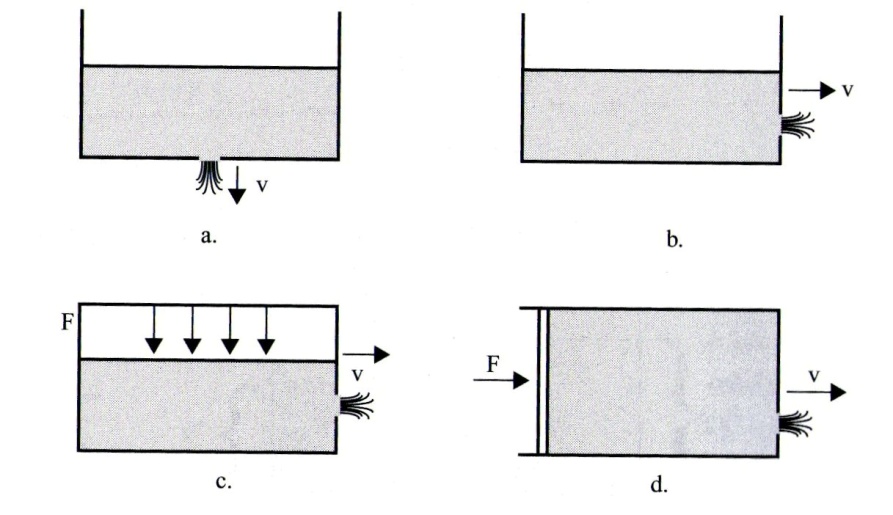
Flow of Liquids from Containers
The speed of the liquid in the outlet depends on several factor as shape, liquid density, position of the outlet in the container, etc. Figure 3 shows some cases with the associated formulas bellow.
a) Base Aperture
Formula
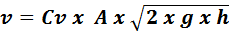
b) Small Lateral Aperture
Formula

c) Excess Pressure on Surface of Liquid
Formula

d) Excess of pressure applied to na outlet point
Formula

Where:
v is the velocity in the outlet
Cv is the velocity coefficient (0.97 for water)
g is the gravity acceleration (9.8 m/s2)
h is the heith of the container filled with water
Pex /? is pressure in excess by area unit